| << Предыдущий параграф |
Распознанный текст, спецсимволы и формулы могут содержать ошибки, поэтому с корректным вариантом рекомендуем ознакомиться на отсканированных изображениях учебника выше
Также, советуем воспользоваться поиском по сайту, мы уверены, что вы сможете найти больше информации по нужной Вам тематике
Аннотированная литература
Нижеперечисленные вводные обзоры и книги содержат обширные списки оригинальной литературы (только в статьях Хеллемана около 300 ссылок), поэтому автор даже не пытался привести здесь все ссылки и просит извинения у тех, чьи работы не указаны. Ссылки и материалы для дальнейшего изучения приводятся отдельно к каждой главе.
Введение
Оригинальные работы и общие источники:
Abraham R.H., Shaw С. D. (1981): Dynamics — The Geometry of Behaviour. Aeriel Press, Santa Cruz.
Арнольд В. И. Малые знаменатели II. Доказательство теоремы А. Н. Колмогорова о сохранении условио-периодических движений при малых изменениях функции Гамильтона. — УМН, 1963, т. 18, вып. 5, с. 13.
Arnold V. I., Avez А. (1968): Ergodic Problems of Classical Mechanics. Benjamin, New York.
Balescu R. (1975): Equilibrium and Nonequilibrium Statistical Mechanics. Wiley, New York.
Campbell D., Rose H. (eds.) (1983), “Proc. of the International Conference on ‘Order in Chaos’ in Los Alamos”, Physica 7D, 1.
Chandrasekhar S. (1961): Hydrodynamic and Hydromagnetic Stability. Clarendon Press, Oxford.
Chirikov В. V. (1980): “A Universal Instability of Many Dimensional Oscillator Systems”, Phys. Rep. 52, 463.
Collet P., Eckmann J. P. (1980): Iterated Maps of the Interval as Dynamical Systems. Birkhauser, Boston.
CoulletP., Tresser J. (1978), “Iterations d’Endomorphismes et Groupe de Renormalisation”, C. R. Hebd. Seances Acad. Sci., Ser. A287, 577; J. Phys. (Paris) Coll. 39, C5—25 (1978).
Cvitanovich P. (ed.) (1984): Universality in Chaos. A reprint selection, Adam Hilger, Bristol.
Eckmann J. P. (1981). “Roads to Turbulence in Dissipative Dynamical Systems”, Rev. Mod. Phys, 53, 643.
Feigenbaum M. J. (1978). “Quantitative Universality for a Class of Nonlinear Transformations”, J. Stat. Phys. 19, 25.
Feigenbaum M. (1980): Universal Behaviour in Nonlinear Systems. Los Alamos Science. Garrido L. (ed.) (1982): Dynamical Systems and Chaos. Lect. Notes in Physics 179, Springer, Berlin — Heidelberg — New York — Tokyo.
Gorel D. G., Roessler О. E. (eds.) (1978), “Bifurcation Theory in Scientific Disciplines”, Ann. N. Y. Acad. Sci. 306.
(см. скан)
(см. скан)
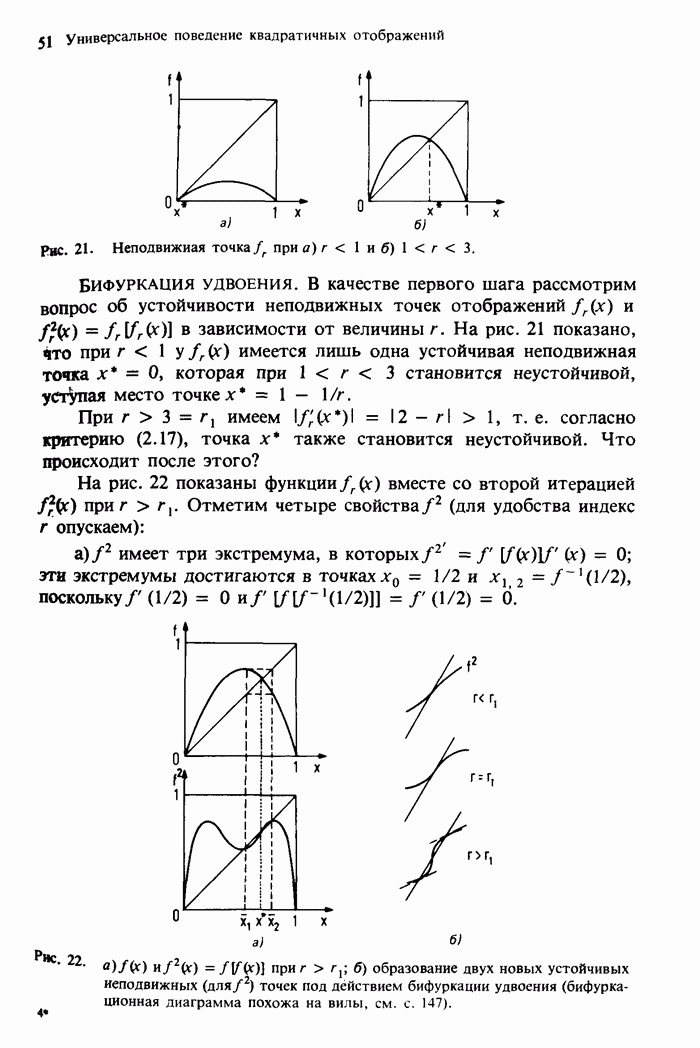
Глава 1. Эксперименты и простые модели
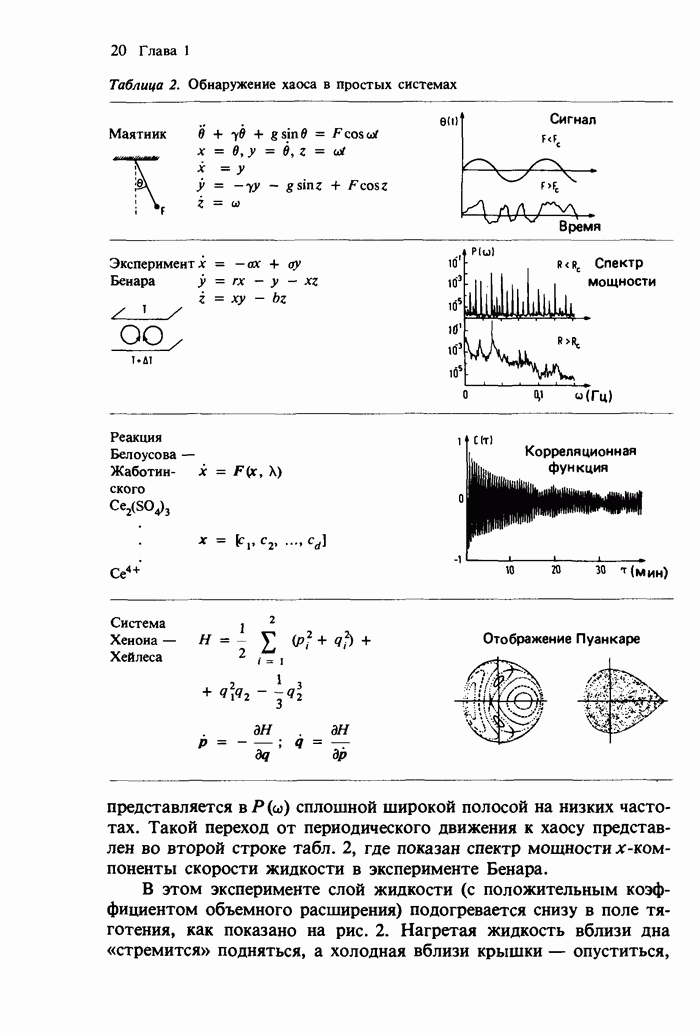
Дополнительную информацию о системах, представленных в табл. 2, можно получить в следующих работах:
Dubois М., Berge Р. (1981), “Instabilites de Couche Limite dans un Fluide en Convection. Evolution vers la Turbulence”, J. Phys. (Paris) 42, 167.
Epstein I. R., Kustin K., de Kepper P., Orban M. (1983), “Oscillating Chemical Reactions”, Sci. Am. 248, No. 3.
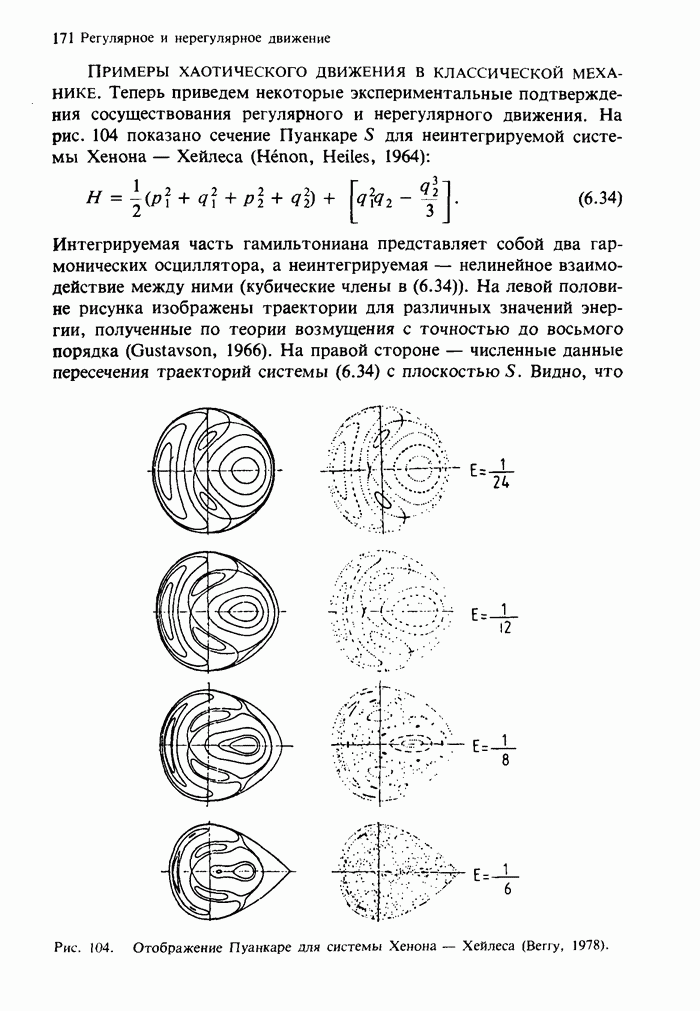
Нёпоп M., Heiles C. (1964), “The Applicability of the Third Integral of the Motion: Some Numerical Results”, Astron. J. 69, 73.
D'Humieres D., Beasly M. R., Huberman B. A., Libchaber A. (1982), “Chaotic States and Routes to Chaos in the Forced Pendulum”, Phys. Rev. A26, 3483.
McLaughlin J. B., Martin P. C. (1975), “Transition to Turbulence of a Statically Stressed Fluid System”, Phys. Rev. A12, 186.
Libchaber A., Maurer J. (1982), In T. Riste (ed.): Nonlinear Phenomena at Phase Transitions and Instabilities. NATO Advanced Study Inst., Plenum Press, New York.
Lorenz E. N. (1963), “Deterministic Nonperiodic Flow”, J. Atmos. Sci. 20, 130.
RouxJ. C., Rossi A., Bachelart S., Vidal C. (1981), “Experimental Observations of Complex Behaviour During a Chemical Reaction”, Physica 2D, 395.
Saltzman B. (1981), “Finite Amplitude Free Convection as an Initial Value Problem 1”, J. Atmos. Sci. 19, 329.
Simoyi R. H., Wolf A., Swinney H. L. (1982), “One-Dimensional Dynamics in a Multicomponent Chemical Reaction”, Phys. Rev. Lett. 49, 245.
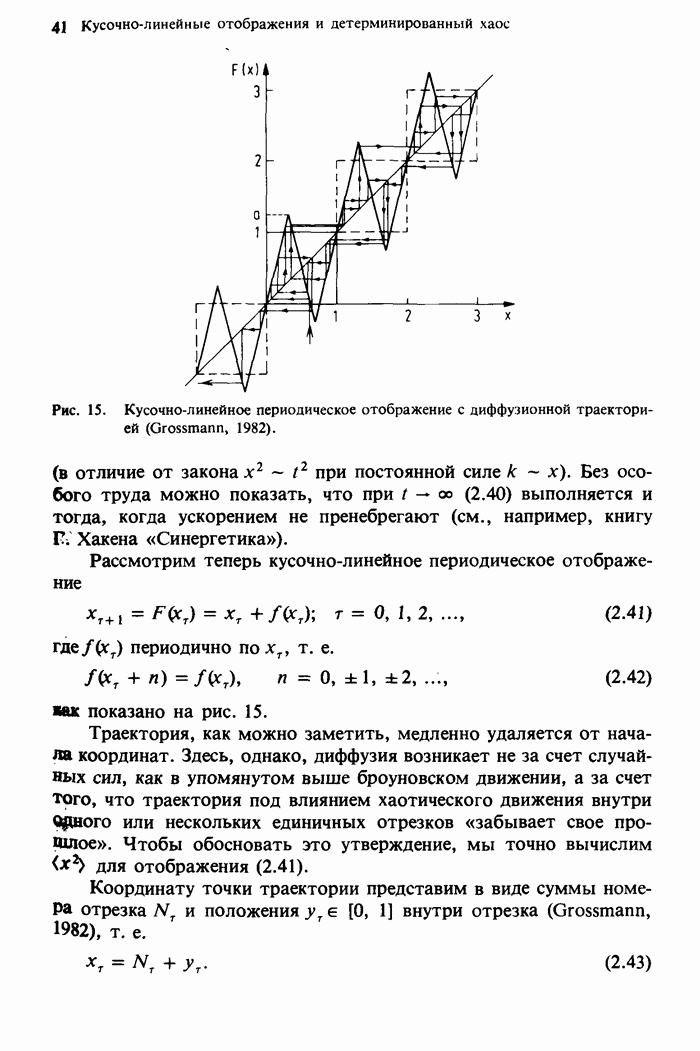
Глава 2. Кусочно-линейные отображения и детерминированный хаос
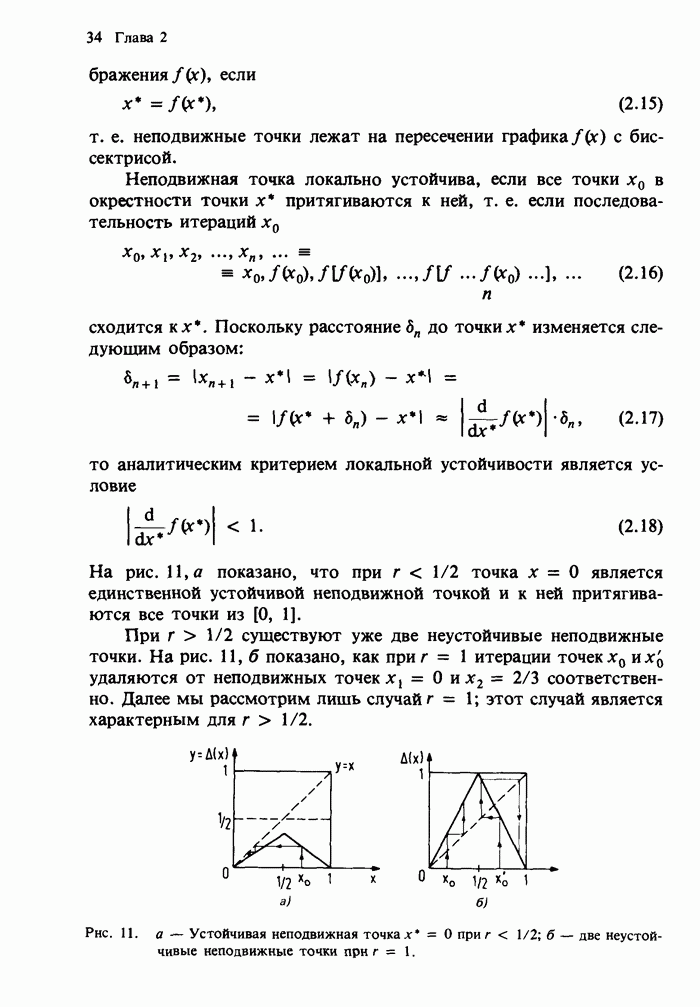
Сдвиг Бернулли обсуждается в работах
Billingsley Р. (1964): Ergodic Theory and Information. Wiley, New York. (Имеется перевод: Биллингслей П. Эргодическая теория и информация. — М.: Мир, 1969.)
Shaw R. S. (1981), in Н. Haken (ed.): Chaos and Order in Nature. Springer, Berlin — Heidelberg — New York — Tokyo.
Доказательство того факта, что двоичное разложение почти всех иррациональных чисел из [0, 1] бесконечное число раз включает в себя любую конечную последовательность, имеется в работе
Hardy G. Н., Wright Е. М. (1938): The Theory of Numbers. Oxford University Press, Oxford, p. 125.
(см. скан)
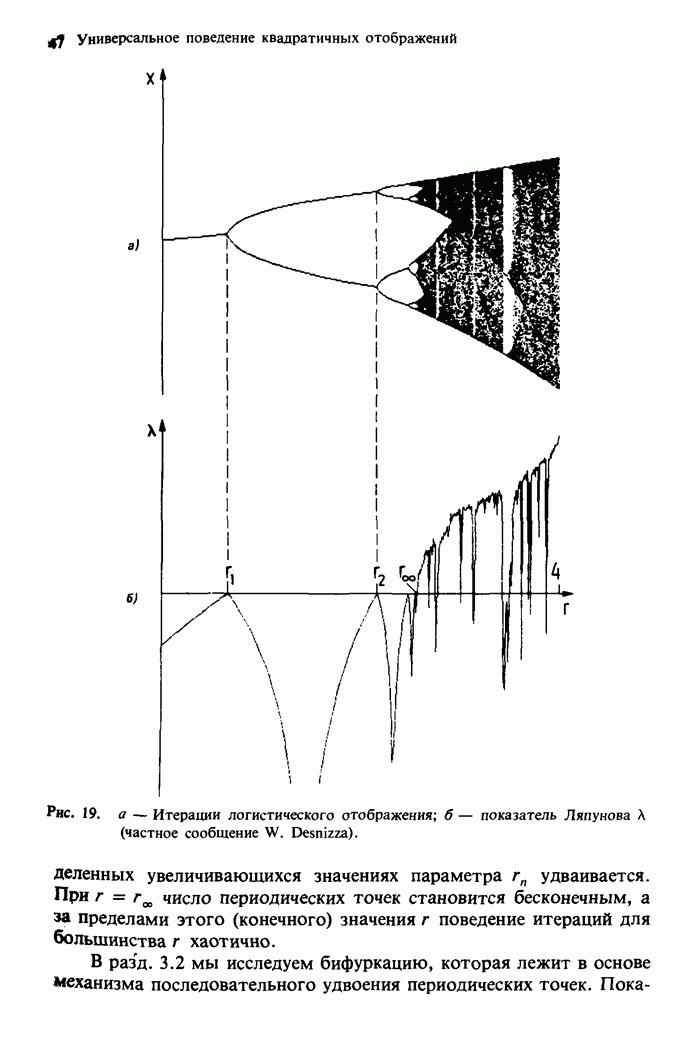
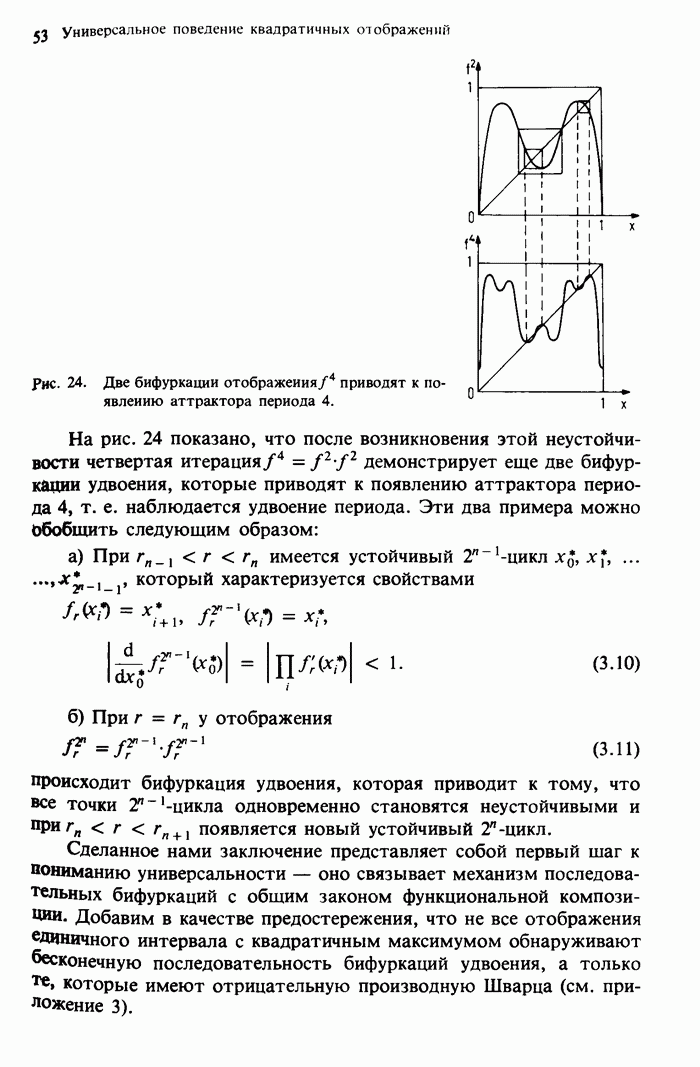
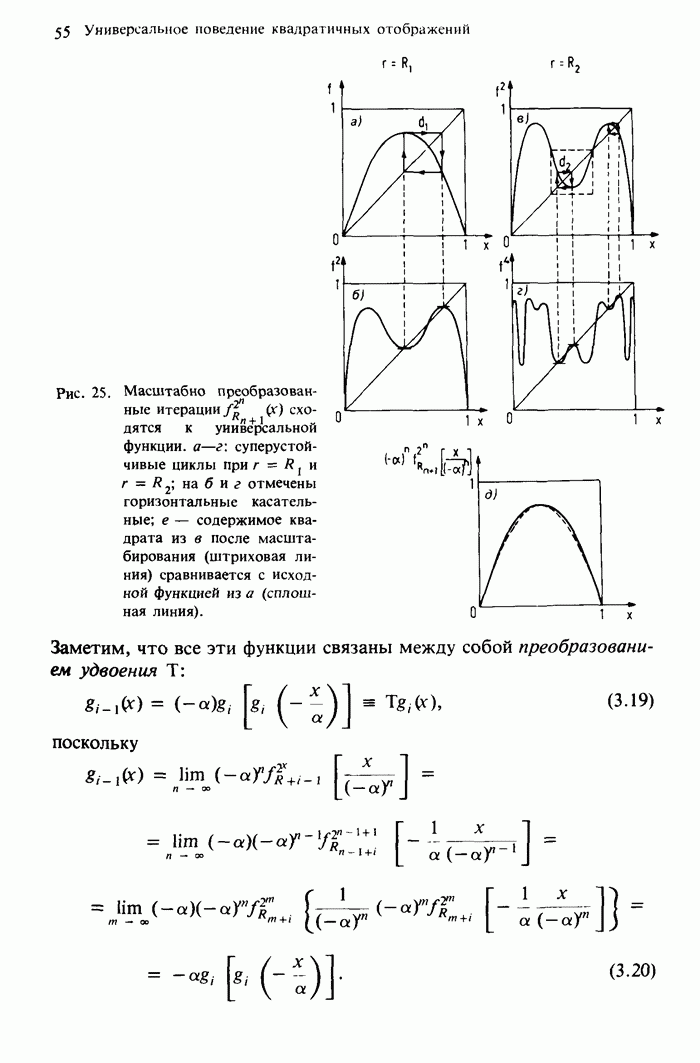
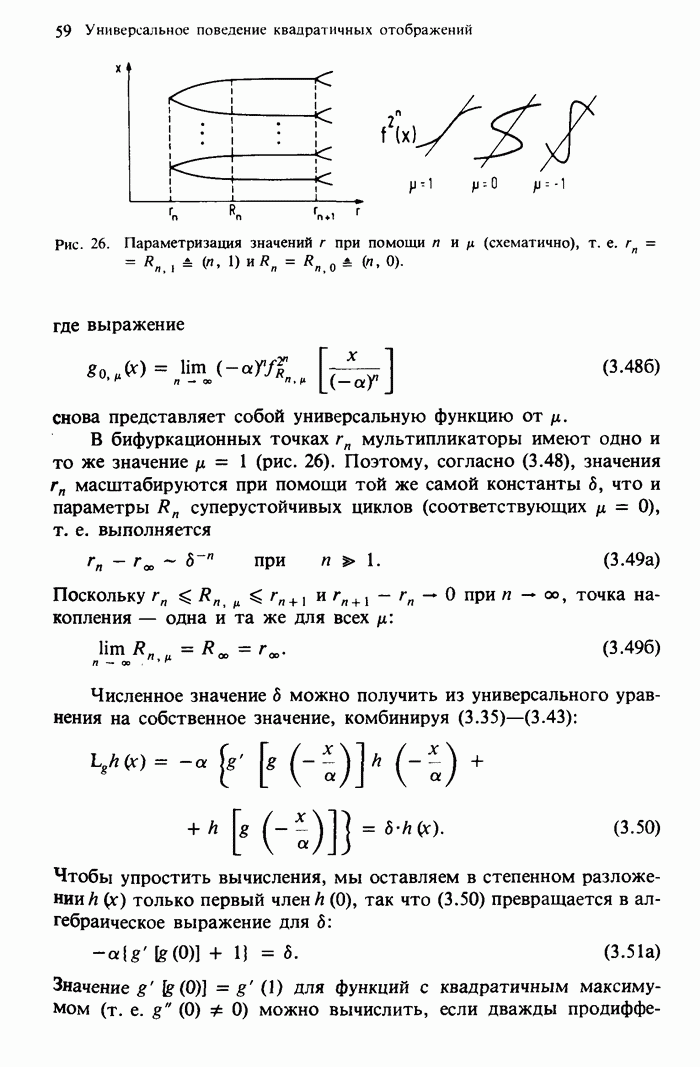
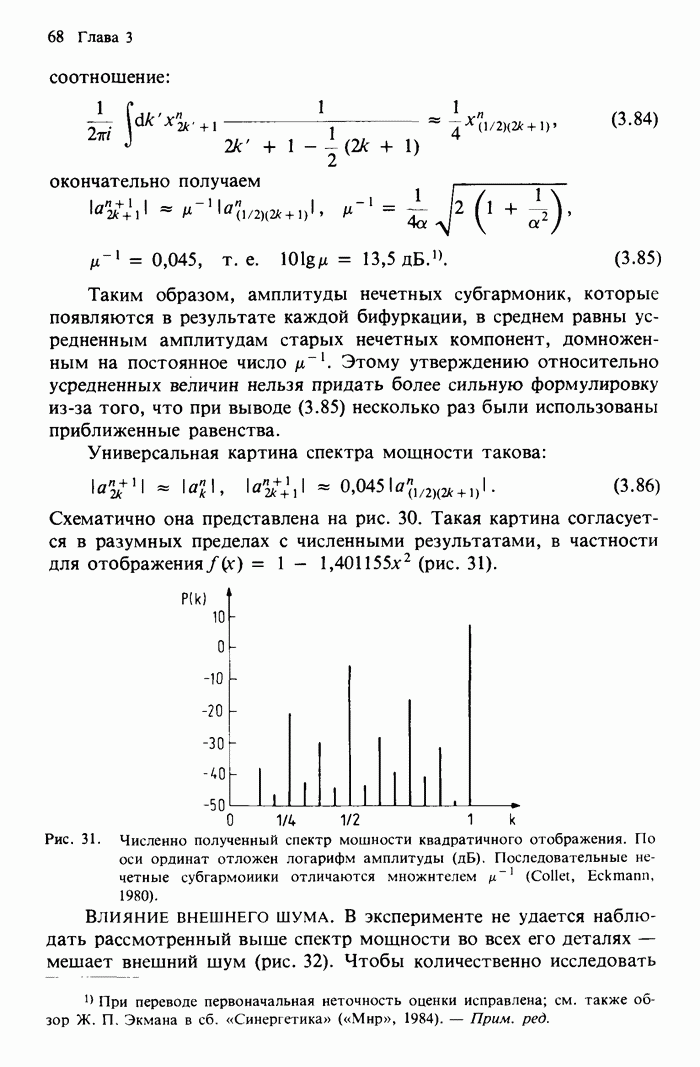
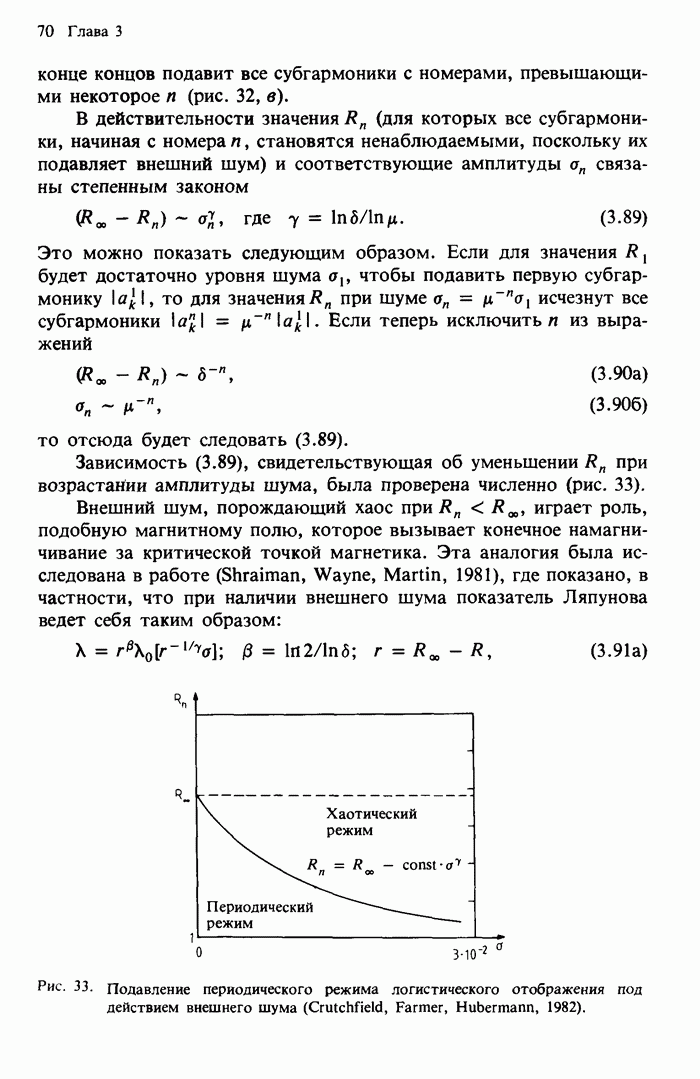
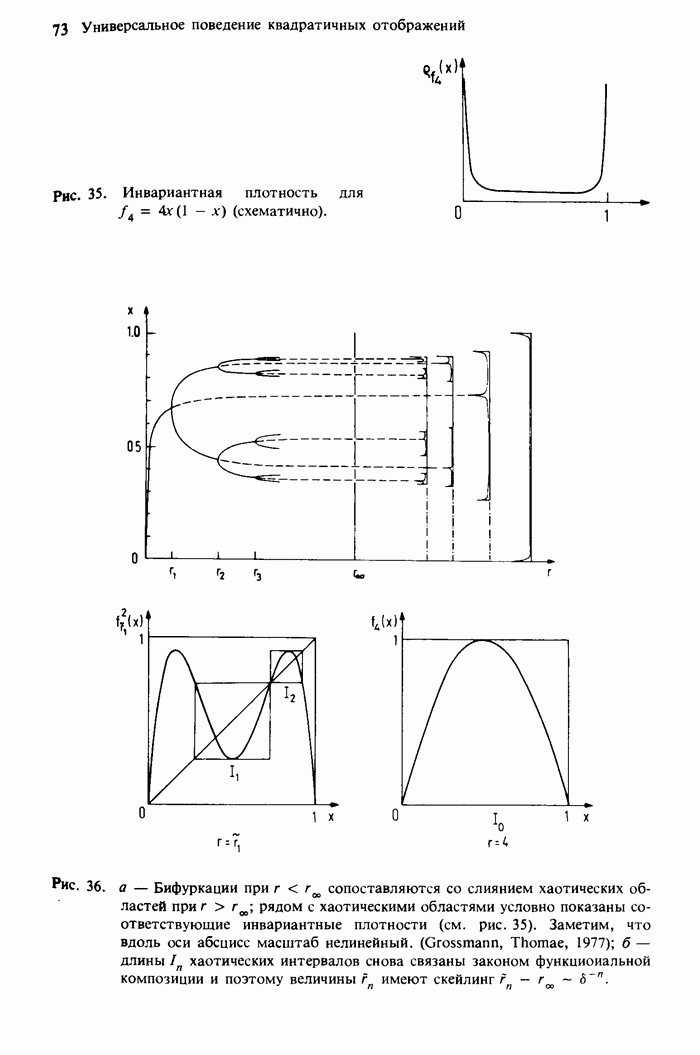
Глава 3. Универсальное поведение квадратичных отображений
Оригинальные статьи и общие сведения
Collet Р., Eckmann J. Р. (1980): Iterated Maps of the Interval as Dynamical Systems. Birkhauser, Boston.
Collet P., Eckmann J. P., Landford О. E. (1980), “Universal Properties of Maps on an Interval”, Commun. Math. Phys. 76, 211.
Collet P., Tresser J. (1978), “Iteration d’Endomorphismes et Groupe de Renormalisation”, C. R. Hebd. Seances Acad. Sci. Series A287, 577; J. Phys. (Paris) C5, 25 (1978). Derrida B., GervoisA., Pomeau Y. (1979), “Universal metric Properties of Bifurcations and Endomorphisms”, J. Phys. 12A, 269.
Feigenbaum M. J. (1978), “Quantitative Universality for a Class of Nonlinear Trasfoma-tions”, J. Stat. Phys. 19, 25.
Feigenbaum M. J. (1979), “The Universal Properties of Nonlinear Transformations”, J. Stat. Phys. 21, 669.
Grossmann S., Thomae S. (1977), “Invariant Distributions and Stationary Correlation Functions of One-Dimensional Discrete Processes”, Z. Naturforsch. 32A, 1353. Hubermann B. A., Rudnick J. (1980), “Scaling Behaviour of Chaotic Flows”, Phys. Rev. Lett. 45, 154.
May R. M. (1976), “Simple Mathematical Models with Very Complicated Dynamics”, Nature 261, 459.
Metropolis M., Stein M. L., Stein P. R. (1973), “On Finite Limit Sets for Transformations of the Unit Interval”, J. Combinatorial Theory (A) 16, 25.
Lanford О. E. (1982): “A Computer Assisted Proof of the Feigenbaum Conjectures”, Bull. Am. Math. Soc. 6, 427.
Peitgen H. O., Richter P. H. (1984): Harmonie in Chaos und Kosmos, und Morphologie komplexer Gr.enzen; Bilder aus der Theorie dynamischer Systeme.
(см. скан)
(см. скан)
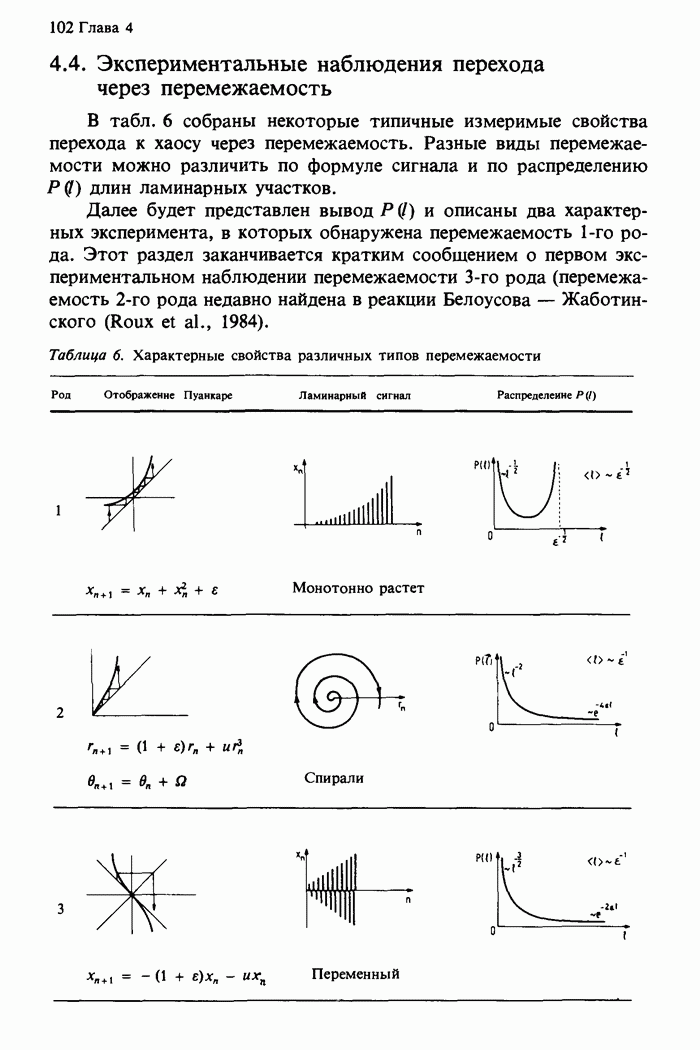
Глава 4. Переход к хаосу через перемежаемость
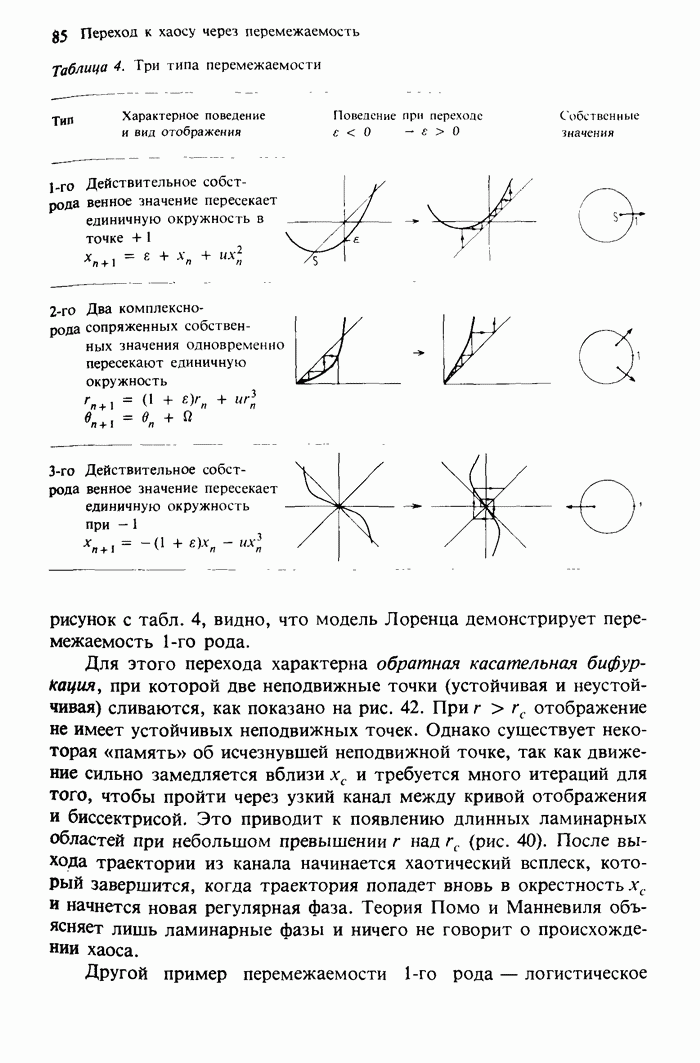
Переход через перемежаемость впервые исследован в работах
Manneville Р., Pomeau Y. (1979), “Intermittency and the Lorenz Model”, Phys. Lett. 75 A, 1.
Manneville P., Pomeau Y. (1980), “Different Ways to Turbulence in Dissipative Dynamical Systems”, Physica ID, 219.
Pomeau Y., Manneville P. (1979), in G. Laval, D. Gresillon (Eds.): Intrinsic Stochasticity in Plasmas. Ed. de Physique, Orsay, p. 239.
Pomeau Y., Manneville P. (1980), “Intermittent Transition to Turbulence in Dissipative Dynamical Systems”, Comm. Math. Phys. 74, 189.
Влияние внешнего шума на перемежаемость
Eckmann J. P., Thomas L., Wittwer P. (1981), “Intermittency in the Presence of Noise”, J. Phys. 14A, 3153.
Hirsch J. E., Hubermann B. A., Scalapino D. J. (1981), “Theory of Intermittency”, Phys. Rev. 25A, 519.
Ренормгрупповой подход к исследованию перемежаемости
Hirsch J. Е., Nauenberg М., Scalapino D. J. (1982), “Intermittency in the Presence of Noise: A Renormalization Group Formulation”, Phys. Lett. 87A, 391.
HuB., Rudnick J. (1982), “Exact Solution of the Feigenbaum Renormalization Group Equations for Intermittency”, Phys. Rev. Lett. 48, 1645.
Фликкер-шум
Ben-Mizrachi A., Procaccia I., Rosenberg N., Schmidt A., Schuster H. G. (1984), “Real and Apparent Divergencies in Low-Frequency Spectra of Nonlinear Dynamical Systems”, Phys. Rev. A.
Dutta P., Horn P. M. (1981), “Low-Frequency Fluctuations in Solids”, Rev. Mod. Phys. 53, 497.
Manneville P. (1980), “Intermittency, Self-Similarity and 1//-Spectrum in Dissipative Dynamical Systems”, J. Phys. (Paris) 41, 1235.
Procaccia I., Schuster H. G. (1983), “Functional Renormalization Group Theory of Universal l//-Noise in Dynamical Systems”, Phys. Rev. 28A, 1210.
Wolf D. (1978): Noise in Physical Systems. Series on Electrophysics 2. Springer, Heidelberg — New York.
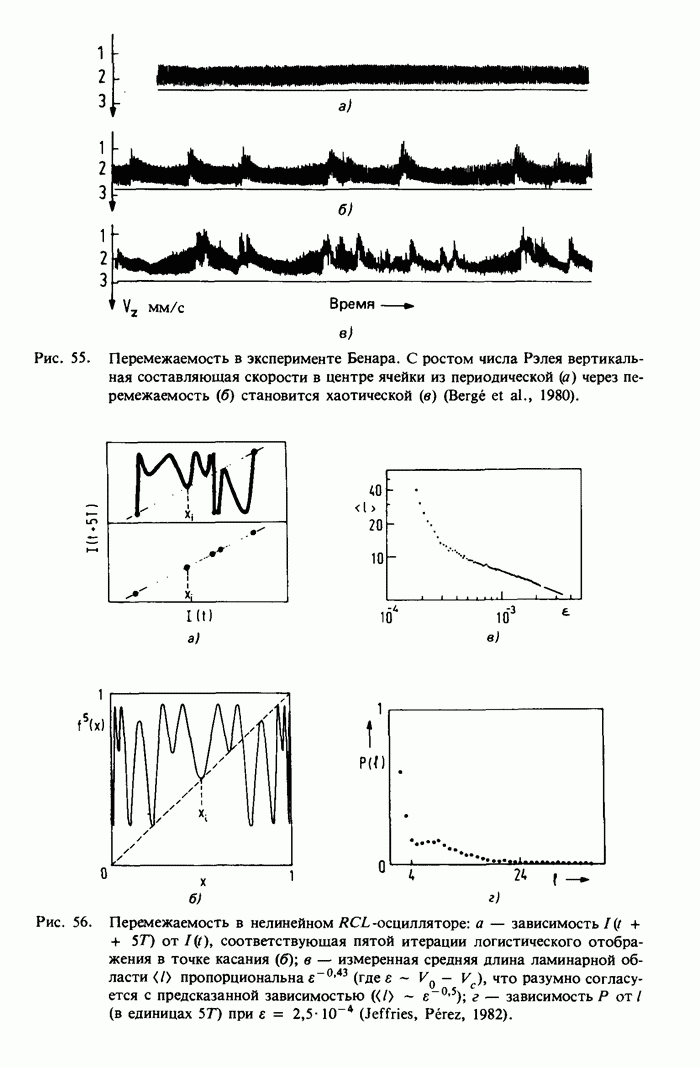
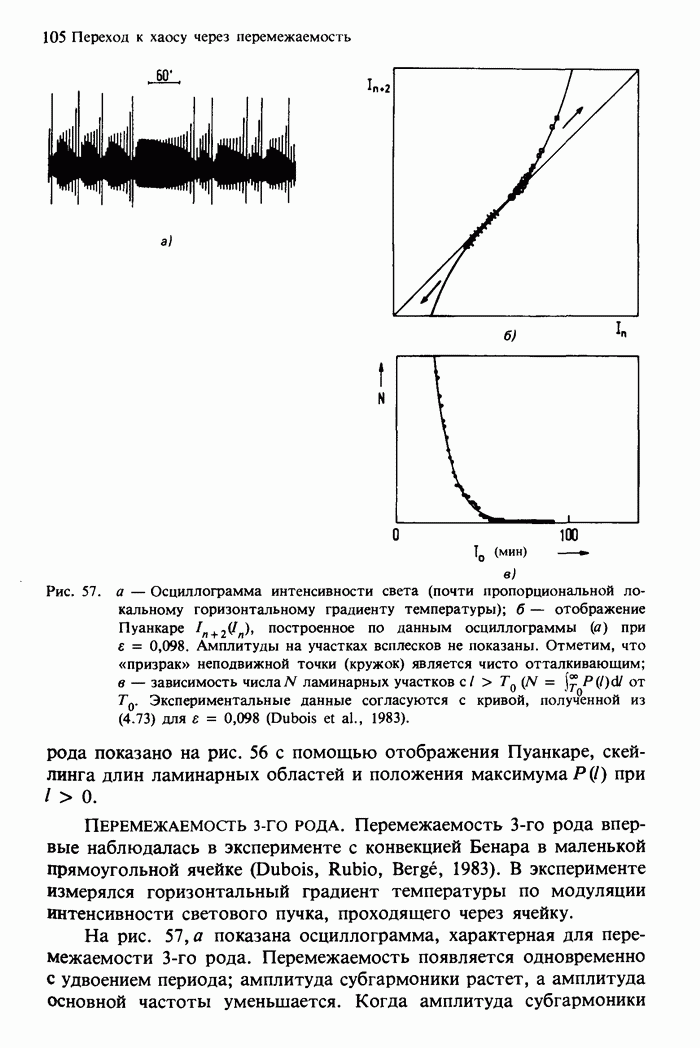
Эксперименты по наблюдению перемежаемости
BergeP., Dubois М., Manneville P., Pomeau Y. (1980), “Intermittency in Rayleigh-Benard Convection”, J. Phys. (Paris) Lett. 41, L-344.
Dubois М., Rubio M. A., BergeP. (1983), “Experimental Evidence of lntermittencies Associated with a Subharmonic Bifurcation”, Phys. Rev. Lett. 51, 1446.
(см. скан)
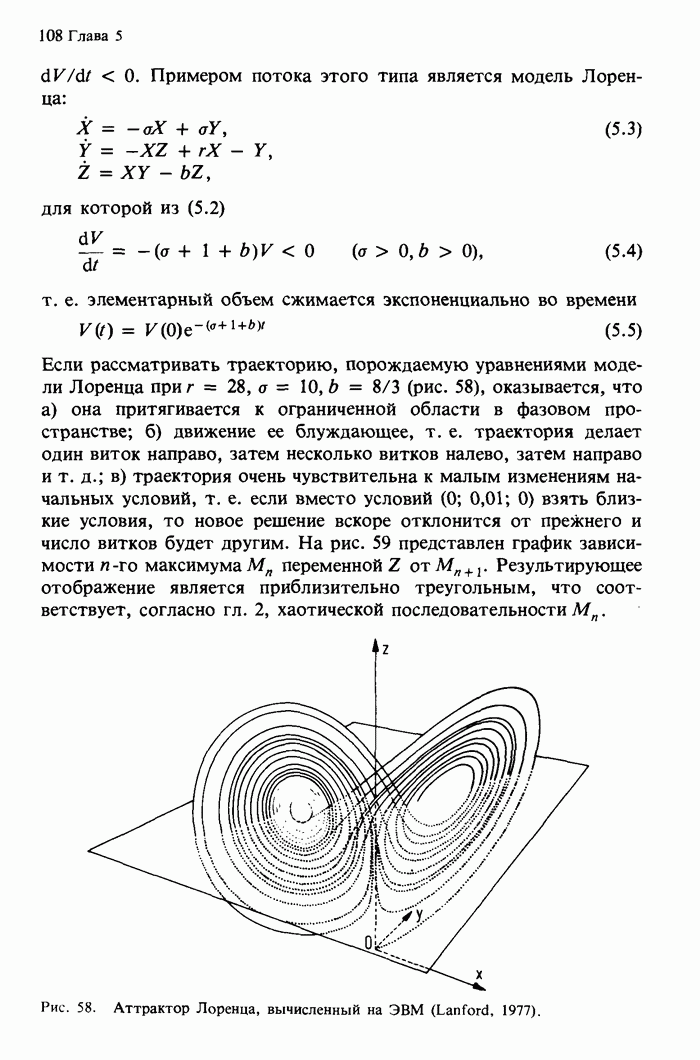
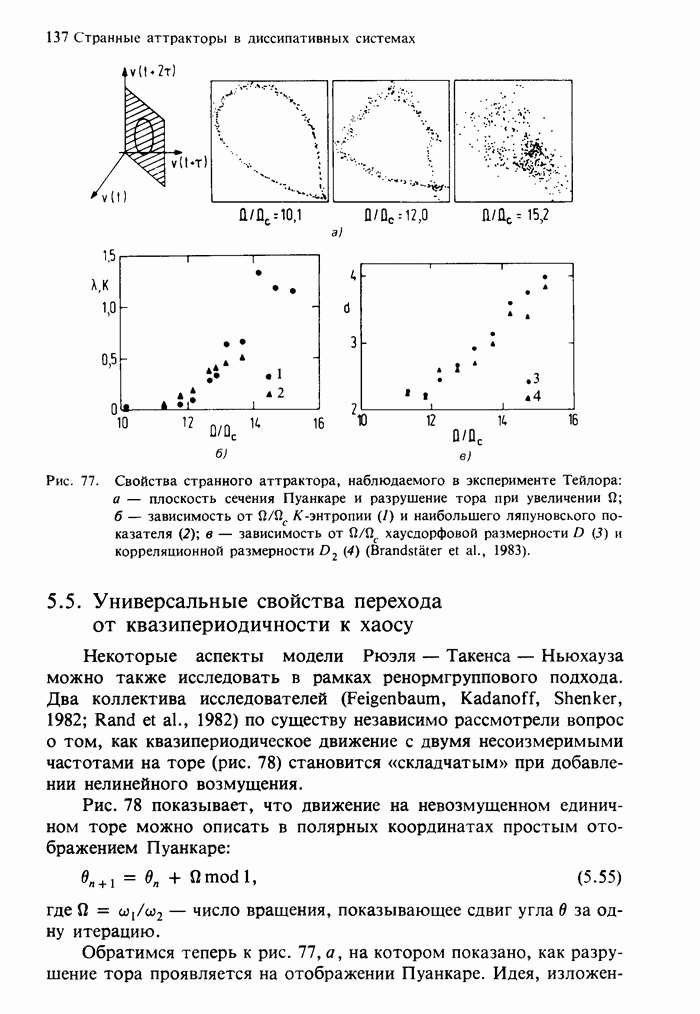
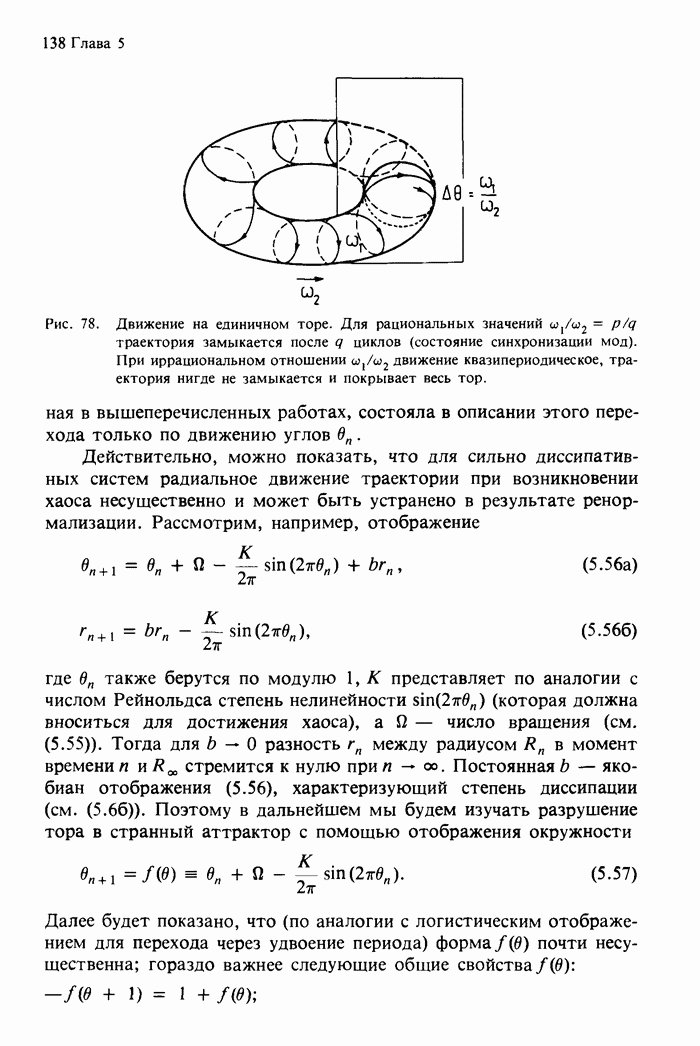
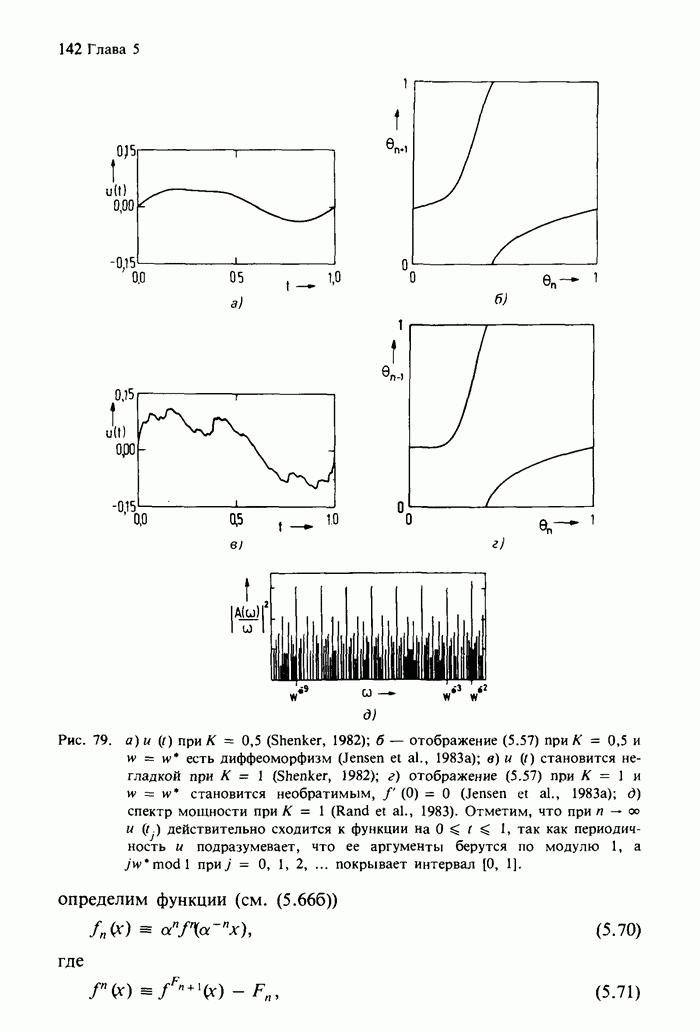
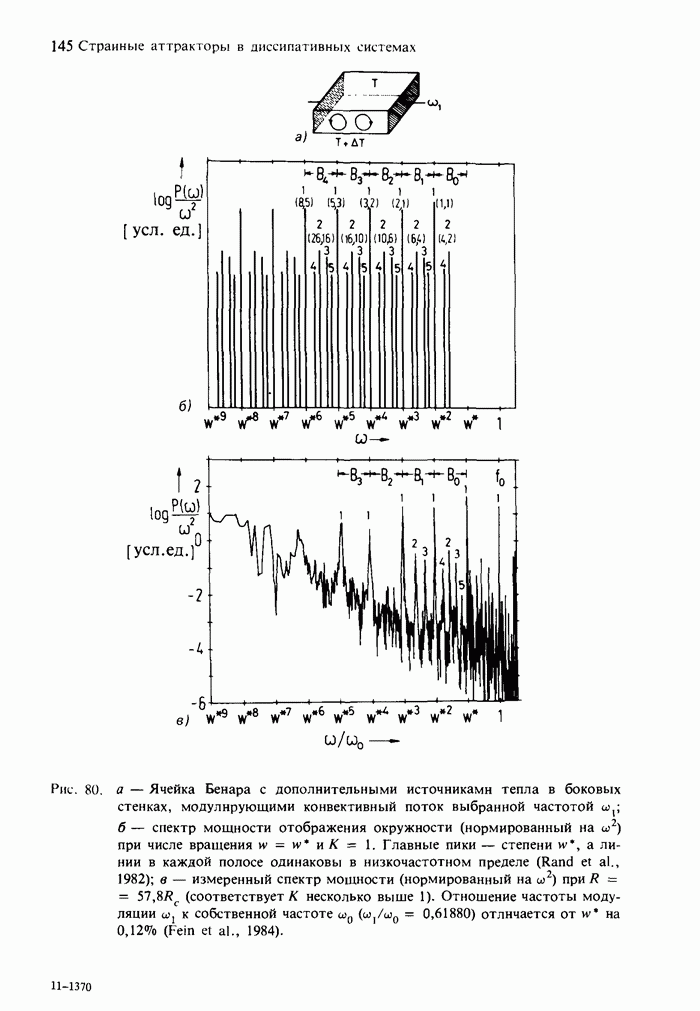
Глава 5. Странные аттракторы в диссипативных динамических системах
Введение и определение странного аттрактора
Eckman J. Р. (1981), “Roads to Turbulence in Dissipative Dynamical Systems”, Rev. Mod. Phys. 53, 643.
Нёпоп M. (1976), “A Two-Dimensional Map with a Strange Attractor”, Commun. Math. Phys. 50, 69.
Lanford О. E. (1977), “Turbulence Seminar”, in P. Bernard, T. Rativ (eds.): Lecture Notes in Mathematics 615, Springer, Heidelberg— New York, p. 114.
Lorenz E. N. (1963), “Deterministic Nonperiodic Flow”, J. Atmos. Sci. 20, 130. Mandelbrot B. (1982): The Fractal Geometry of Nature, Freeman, San Francisco.
OttE. (1981), “Strange Attractors and Chaotic Motions of Dynamical Systems”, Rev.
Mod. Phys. 53, 655.
Ruelle D. (1980), “Strange Attractors”, Math. Intelligencer 2, 126.
Доказательство теоремы Пуанкаре — Бендикссона
Hirsch М. W., Smale S. (1965): Differential Equations, Dynamic Systems and Linear Algebra. Academic Press, New York.
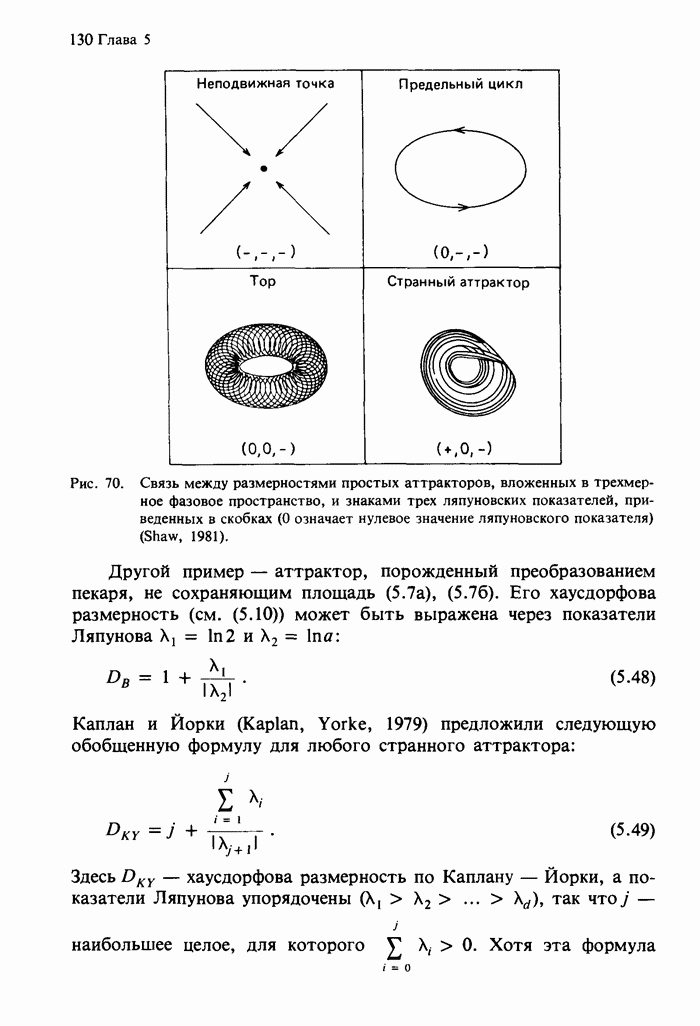
Преобразование пекаря (сохраняющее площадь и диссипативное) и гипотеза Каплана — Йорки обсуждаются в работах
Kaplan J., Yorke J. (1979), in H. О. Peitgen, Н. О. Walther (eds.): Functional Differential Equations and Approximation of Fixed Points. Springer, Heidelberg — New York. LebowitzJ. L., Penrose О. (1973), “Modern Ergodic Theory”, Phys. Today 23 (2). Russel D. A., Hansen J. D., Ott E. (1980), “Dimension of Strange Attractors”, Phys. Rev. Lett. 45, 1175.
Shaw R. S. (1981), “Strange Attractors, Chaotic Behaviour and Information Flow”, Z. Naturforsch. 36a, 80.
Энтропия Колмогорова и связанные с ней вопросы
Arnold V. /., AvezA. (1974): Ergodic Problems of Classical Mechanics. Benjamin, New York.
(см. скан)
(см. скан)
(см. скан)
Глава 6. Регулярное и нерегулярное движение в консервативных системах
Кроме классических трудов (Poincare, 1892; Arnold, Avez, 1968; Moser, 1973; Balescu, 1975), которые вместе с работой (Lorenz, 1963) по диссипативным системам вошли в список литературы к «Введению», имеются также другие прекрасные обзоры и монографии по нерегулярному движению в гамильтоновых системах:
(см. скан)
(см. скан)
Глава 7. Хаос в квантовых системах?
Вопрос о квантовании классических неинтегрируемых систем восходит к работе
Einstein А. (1917): “Zum Quantensatz von Sommerfeld und Epstein”, Vehr. Dtsch. Phys. Ges. 19, 82.
Имеются прекрасные обзоры о проблеме стохастичности в квантовых системах:
Berry М. V. (1983), in R.H.G. Hellemann and G. loos (eds.): Chaotic Behaviour of Deterministic Systems. Les Houches Summer School 1981. North-Holland, Amsterdam. Casati G. (1982), in H. Haken (ed.): Evolution of Order and Chaos. Springer, Heidelberg — New York.
Chirikov В. V. (1979): “A Universal Instability of Many-Dimensional Oscillator Systems”, Phys. Rep. 52, 463.
Zaslavski G. M. (1981): “Stochasticity in Quantum Systems”, Phys. Rep. 80, 157.
(см. скан)
(см. скан)
Заключительные замечания
Общие вопросы
Abraham N. В., Gollub J. P., Swinney H. L. (1984), “Testing Nonlinear Dynamics”, Physica 1 ID, 252.
Benedek G., Bilz H., Zeyher R. (1984), “Statics and Dynamics of Nonlinear Systems”, Springer Series in Solid-State Sciences 47, Springer, Berlin — Heidelberg — New York. Berry М. V. (1978), in S. Yorna (ed.): “Topics in Nonlinear Dynamics”. Am. Inst. Phys. Conf. Proc., Vol. 46.
Связанные системы
Bishop A. R., FesserK., Lomdahl P. S., Kerr W. C., Trullinger S. E., Williams М. B. (1983), “Coherent Spatial Structures Versus Time Chaos in a Perturbed Sine-Gordon System”, Phys. Rev. Lett. 50, 1095.
Kuramoto Y. (1977), “Chemical Waves and Chemical Turbulence”, in H. Haken (ed.): Synergetics. Springer, Berlin — Heidelberg — New York.
Vidal C., Pacault A. (1981): Nonlinear Phenomena in Chemical Dynamics. Springer, Berlin — Heidelberg — New York.
Winfree A. T. (1980), “The Geometry of Biological Time”, Biomathematics 8, Springer, Berlin — Heidelberg — New York.
Турбулентность
Ruelle D. (1983), “Five Turbulent Problems”, Physica 7D, 40.
Квантовые системы
Graham R. (1984), “Chaos in Lasers”, in E. Frehland (ed.): Synergetics — From Microscopic to Macroscopic Order. Springer, Berlin — Heidelberg — New York.
Elgin J- M, Sarkar S. (1984), “Quantum Fluctuations and the Lorenz Strange Attractor”, Phys. Rev. Lett. 52, 1215.
MisraB., Prigogine I. (1980): “On the Foundations of Kinetic Theory”, Suppl. Progr. Theor. Physics 69, 101.
Случайные числа
De Long H. (1970): Randomness and Godel’s Incompleteness Theorem. Addison-Wesley, Reading, MA.
Биология
Hess В., Markus M. (1984), “Time Pattern Transitions in Biochemical Processes”, in E. Frehland (ed.): Synergetics — From Microscopic to Macroscopic Order. Springer, Berlin — Heidelberg — New York.
Schuster P. (ed.) (1984), “Stochastic Phenomena and Chaotic Behaviour in Complex Systems”, Springer Series in Synergetics 21, Springer, Berlin— Heidelberg— New York.
| << Предыдущий параграф |
- Предисловие редакторов перевода
- Литература
- Предисловие
- Введение
- 1. Эксперименты и простые модели
- 1.1. Экспериментальное обнаружение детерминированного хаоса
- 1.2. Ротатор, возбуждаемый периодическими толчками
- 2. Кусочно-линейные отображения и детерминированный хаос
- 2.2. Характеристики хаотического движения
- 2.3. Детерминированная диффузия
- 3. Универсальное поведение квадратичных отображений
- 3.1. Параметрическая зависимость итераций
- 3.2. Бифуркация удвоения и преобразование удвоения
- 3.3. Самоподобие, универсальный спектр мощности и влияние внешнего шума
- 3.4. Аналогия между удвоением периода и фазовыми переходами
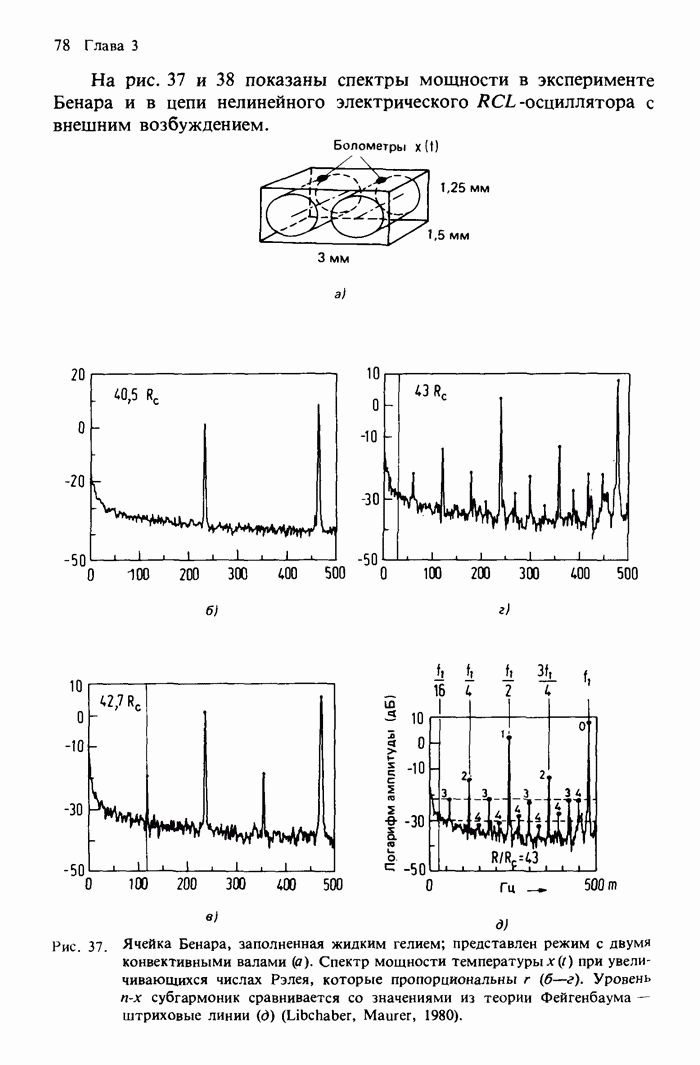
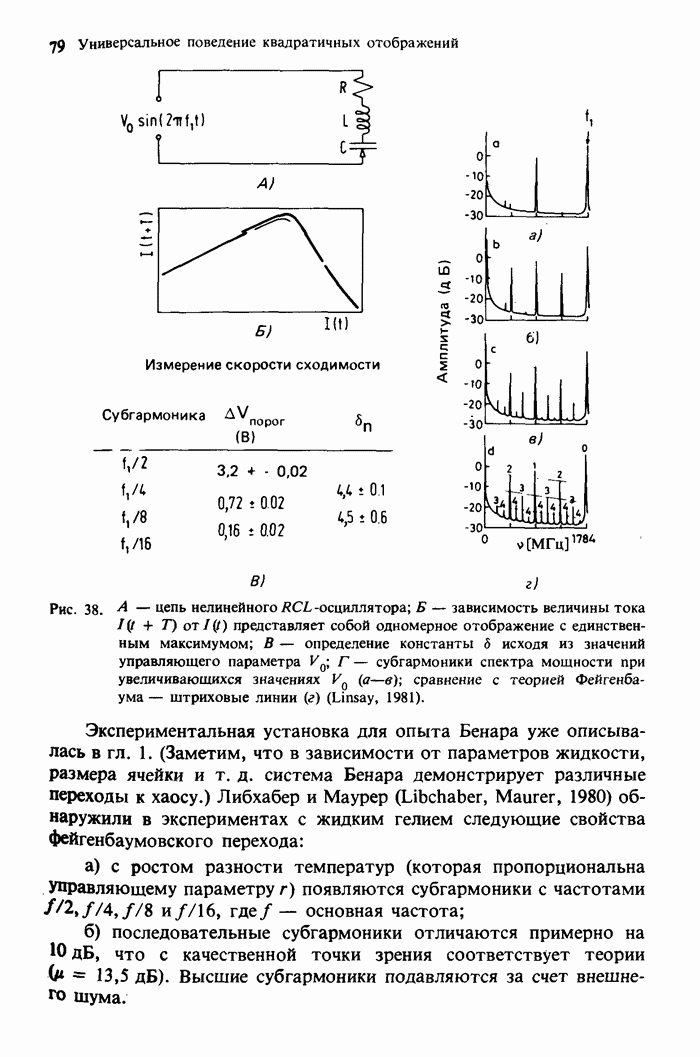
- 3.5. Экспериментальное подтверждение бифуркационного перехода
- 4. Переход к хаосу через перемежаемость
- 4.2. Ренормгрупповое исследование перемежаемости
- 4.3. Перемежаемость и фликкер-шум
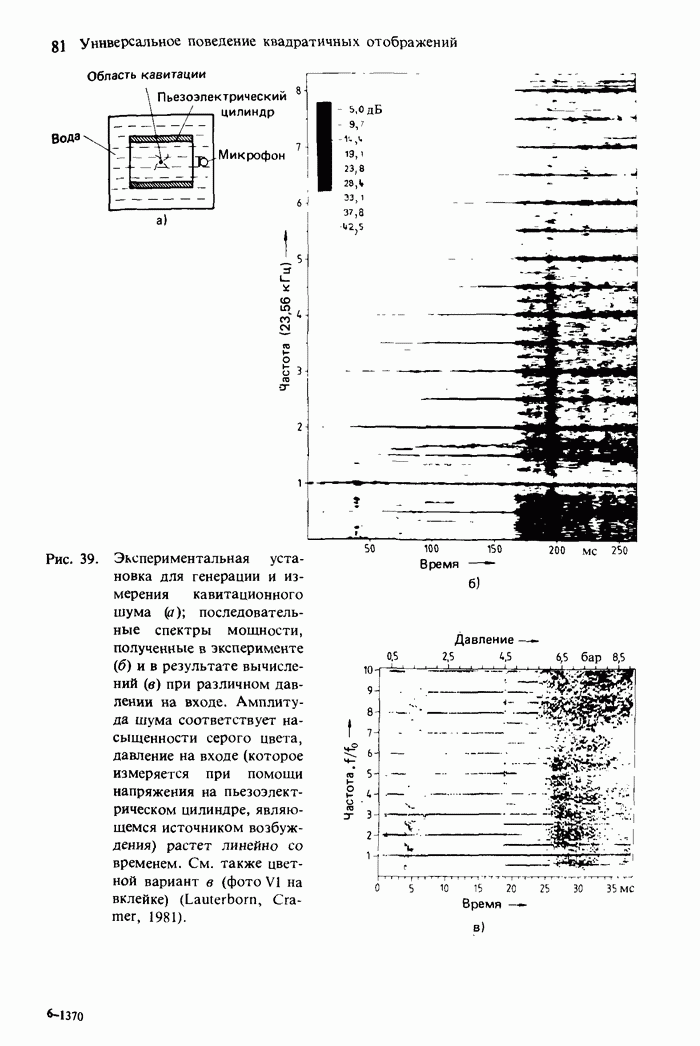
- 4.4. Экспериментальные наблюдения перехода через перемежаемость
- 5. Странные аттракторы в диссипативных динамических системах
- 5.2. Энтропия Колмогорова
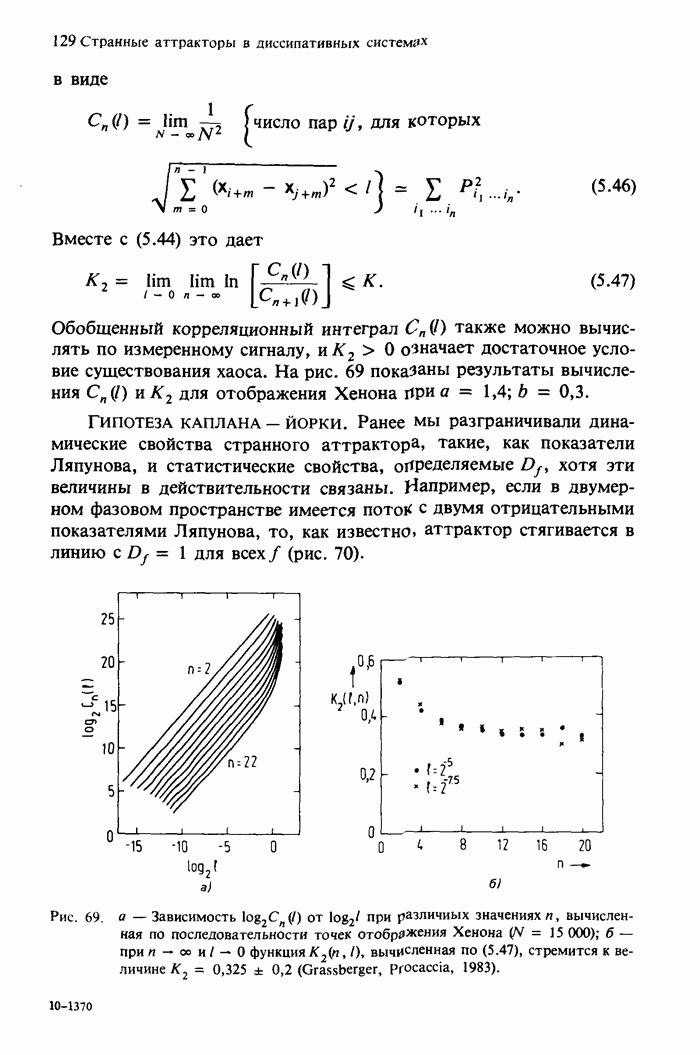
- 5.3. Описание аттрактора по измеренному сигналу
- 5.4. Странные аттракторы и возникновение турбулентности
- 5.5. Универсальные свойства перехода от квазипериодичности к хаосу
- 5.6. Пути перехода к хаосу
- 5.7. Изображения странных аттракторов и фрактальных границ
- 6. Регулярное и нерегулярное движение в консервативных системах
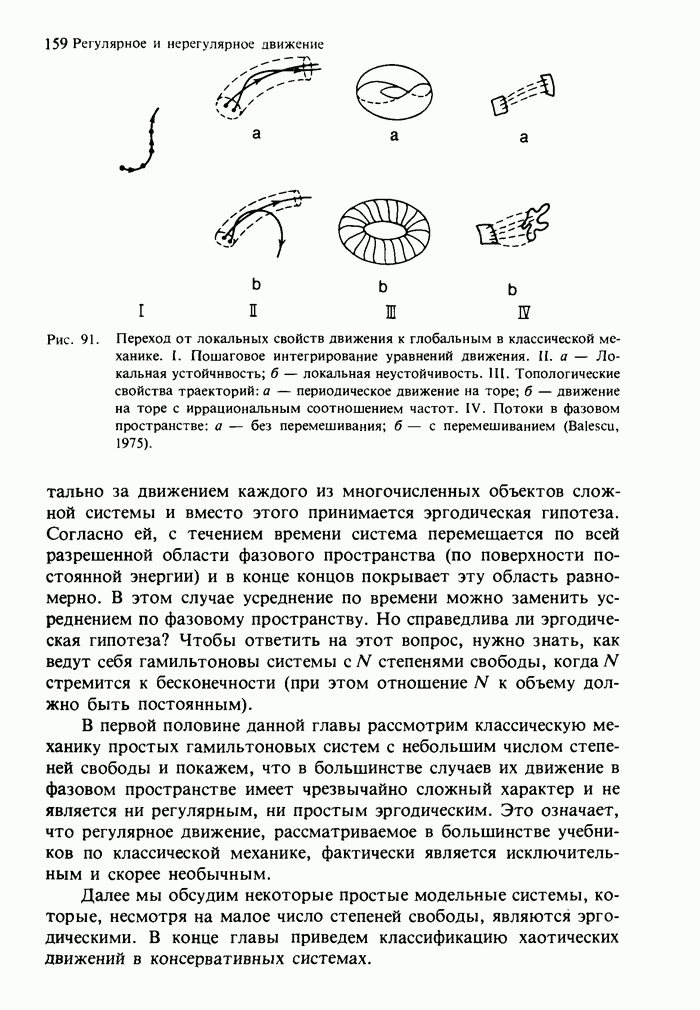
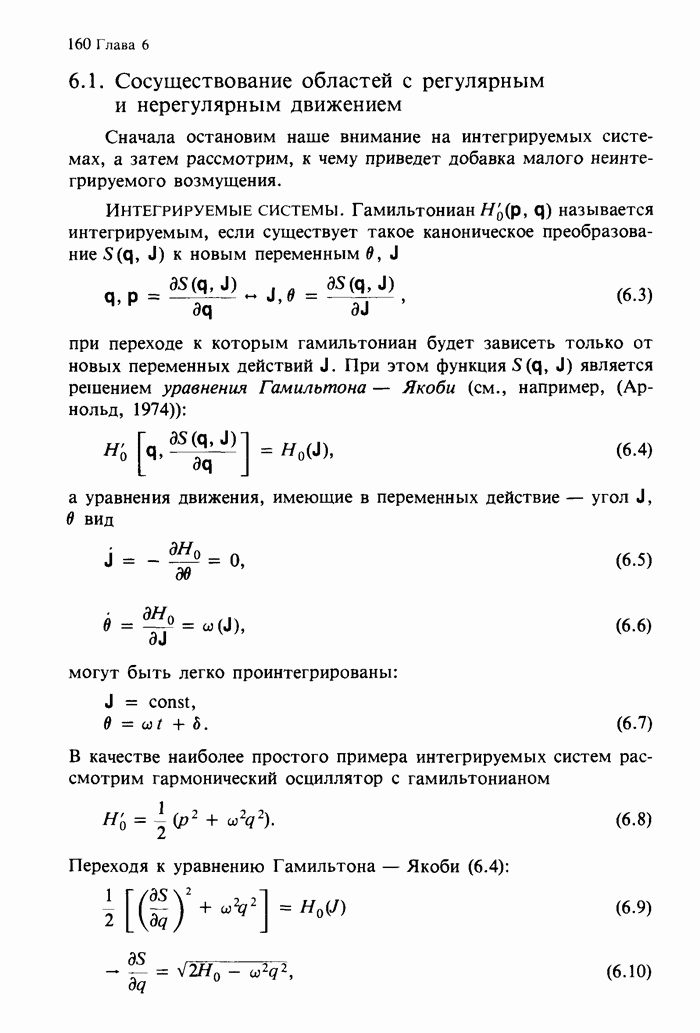
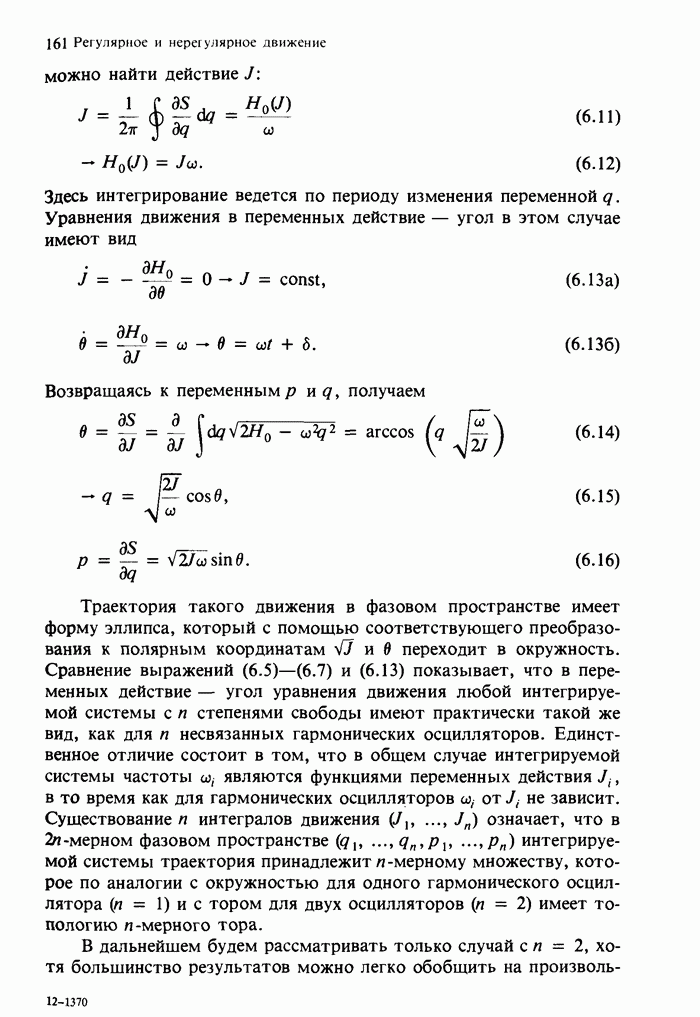
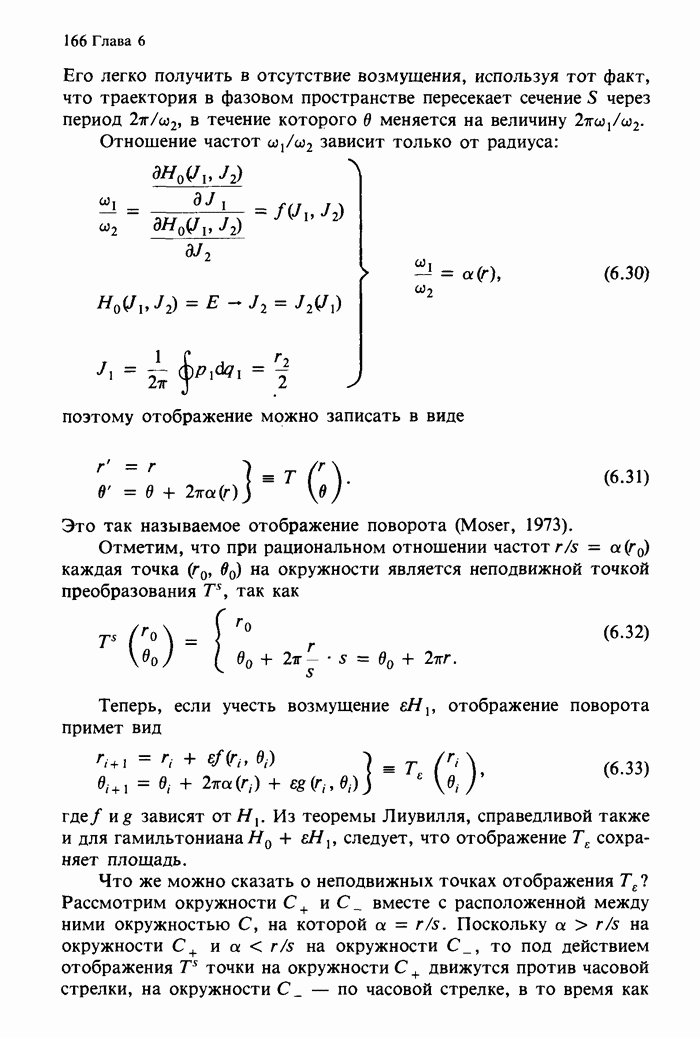
- 6.1. Сосуществование областей с регулярным и нерегулярным движением
- 6.2. Полностью нерегулярное движение и эргодичность
- 7. Хаос в квантовых системах?
- 7.1. Квантовое отображение Арнольда
- 7.2. Квантовая частица в стадионе
- 7.3. Квантовый ротатор с периодическими толчками
- Заключительные замечания
- Приложения
- 1. Вывод модели Лоренца
- 2. Анализ устойчивости, возникновения конвекции и турбулентности в модели Лоренца
- 3. Производная Шварца
- 4. Ренормализация одномерной модели Изинга
- 5. Прореживание и интегралы по траектории для внешнего шума
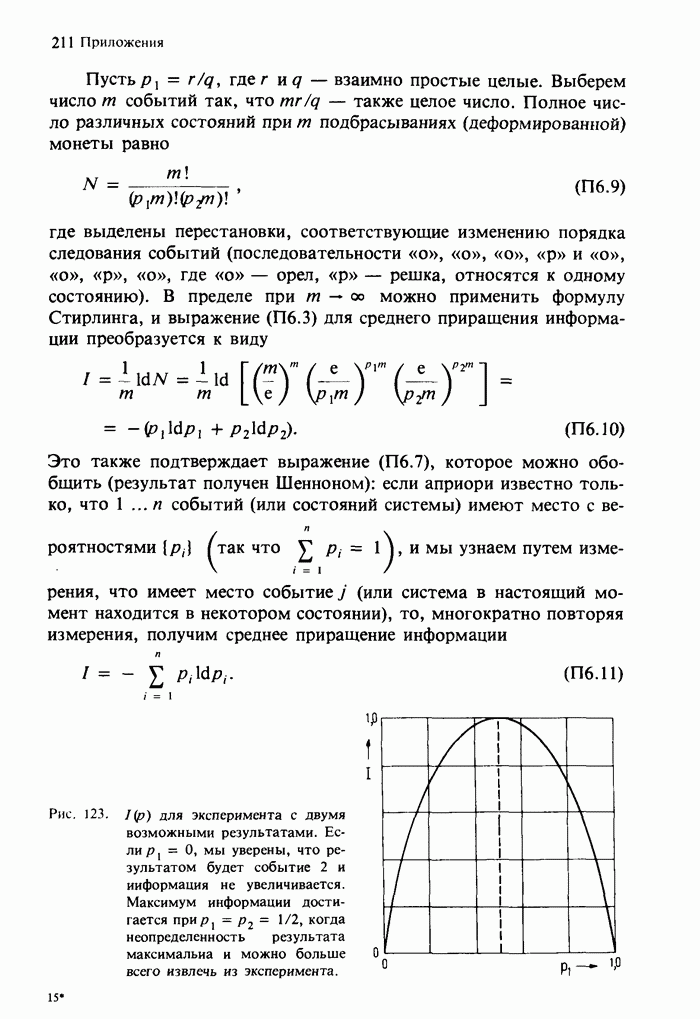
- 6. Мера информации Шеннона
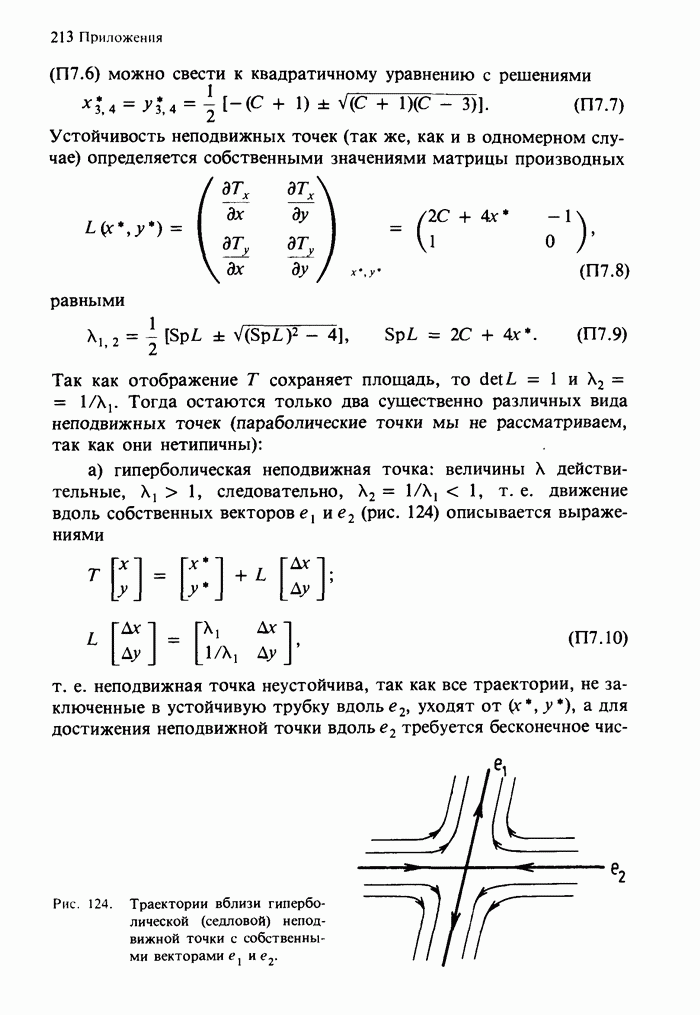
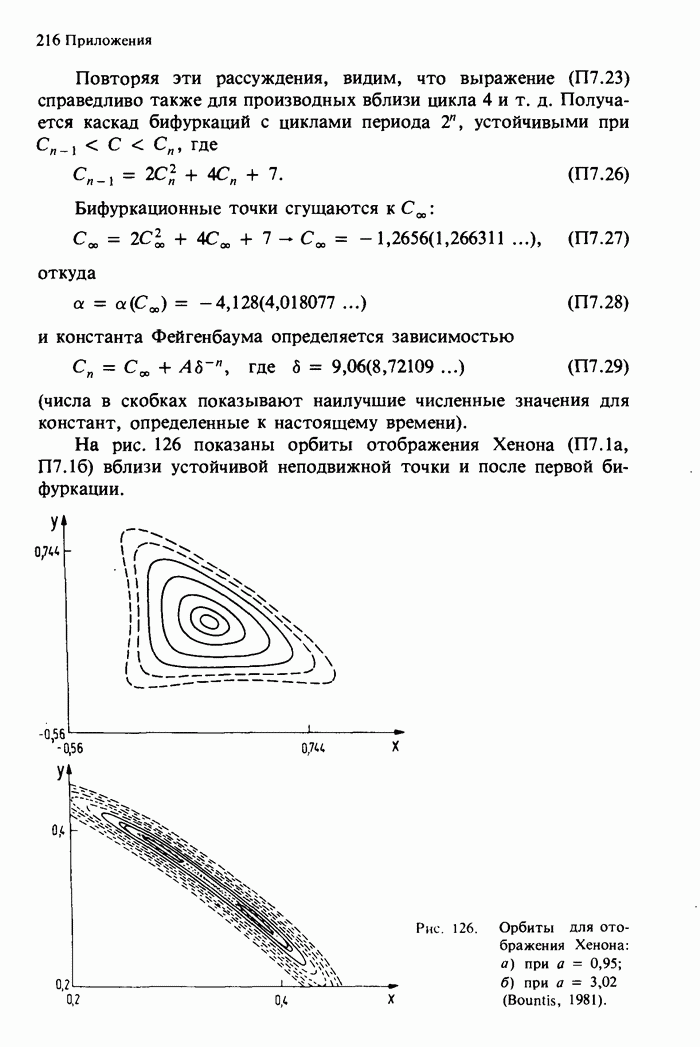
- 7. Удвоение периода для консервативного отображения Хенона
- Аннотированная литература